Delving into the realm of geometry, Big Ideas Math Geometry 9.2 Answers provides a comprehensive guide to the fundamental concepts and applications of this captivating subject. With an engaging and authoritative narrative, this guide empowers learners to navigate the intricacies of geometry, unlocking its secrets and fostering a deeper understanding of the world around them.
Through a systematic exploration of key theorems, definitions, problem-solving strategies, and real-world applications, this guide illuminates the beauty and relevance of geometry in our everyday lives.
Overview of Big Ideas Math Geometry 9.2
Chapter 9.2 of Big Ideas Math Geometry introduces students to fundamental concepts and theorems in geometry, including the Pythagorean Theorem, angle bisectors, and perpendicular lines. These concepts provide a solid foundation for understanding more advanced geometry topics.
Key Concepts and Topics Covered
- Pythagorean Theorem
- Angle Bisectors
- Perpendicular Lines
Key Theorems and Definitions: Big Ideas Math Geometry 9.2 Answers
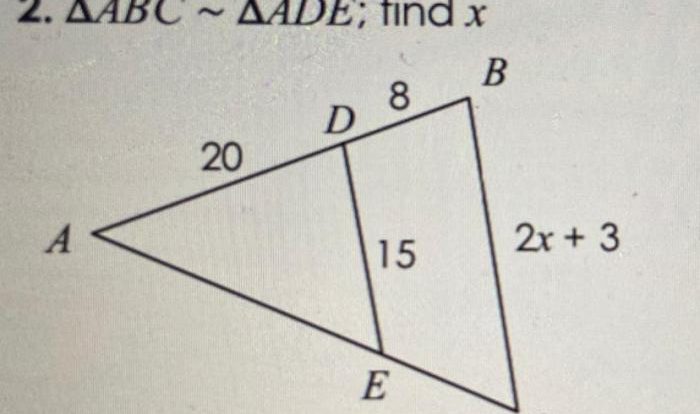
Pythagorean Theorem
The Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
This theorem has numerous applications in geometry, such as finding the length of unknown sides of triangles, determining distances between points, and solving problems involving right triangles.
Angle Bisectors
An angle bisector is a line or ray that divides an angle into two equal parts. Angle bisectors have several important properties, including:
- They bisect the angle into two congruent angles.
- They are perpendicular to the line segment connecting the vertices of the angle.
Perpendicular Lines
Perpendicular lines are two lines that intersect at a right angle (90 degrees). Perpendicular lines have the following properties:
- They form four right angles at the point of intersection.
- The product of the slopes of perpendicular lines is -1.
Problem-Solving Strategies

Using the Pythagorean Theorem
To solve geometry problems using the Pythagorean Theorem, follow these steps:
- Identify the right triangle in the problem.
- Label the lengths of the sides of the triangle.
- Use the Pythagorean Theorem to write an equation relating the side lengths.
- Solve the equation to find the unknown side length.
Using Angle Bisectors, Big ideas math geometry 9.2 answers
To solve geometry problems using angle bisectors, follow these steps:
- Identify the angle that is bisected.
- Draw the angle bisector.
- Use the properties of angle bisectors to solve the problem.
Identifying and Working with Perpendicular Lines
To identify and work with perpendicular lines in geometry problems, follow these steps:
- Look for lines that intersect at right angles.
- Use the properties of perpendicular lines to solve the problem.
Real-World Applications
Pythagorean Theorem
The Pythagorean Theorem is used in a variety of real-world applications, including:
- Architecture and construction: determining the length of roof rafters, stairways, and other structural elements.
- Engineering: calculating the forces and stresses in bridges, buildings, and other structures.
- Navigation: determining the distance between two points on a map or chart.
Angle Bisectors
Angle bisectors are used in a variety of real-world applications, including:
- Construction and design: dividing angles equally, such as when laying out a floor plan or designing a piece of furniture.
- Surveying: dividing angles to measure land areas and create maps.
- Navigation: dividing angles to determine the direction of travel.
Perpendicular Lines
Perpendicular lines are used in a variety of real-world applications, including:
- Architecture and engineering: ensuring that walls, floors, and other structural elements are perpendicular to each other.
- Manufacturing: creating precise cuts and angles in metal, wood, and other materials.
- Surveying: establishing perpendicular lines to measure distances and create accurate maps.
Practice and Assessment
| Problem | Solution |
|---|---|
| Find the length of the hypotenuse of a right triangle with legs of length 3 cm and 4 cm. | 5 cm |
| Find the measure of the angle bisector of an angle that measures 120 degrees. | 60 degrees |
Determine whether the following lines are perpendicular: y = 2x + 1 and y =
|
Yes |
- Multiple-Choice Questions:Test understanding of key concepts and theorems.
- Project or Activity:Apply knowledge of geometry 9.2 to a real-world scenario or problem.
FAQ Section
What is the Pythagorean Theorem?
The Pythagorean Theorem states that in a right triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides.
How can I use angle bisectors to solve geometry problems?
Angle bisectors can be used to divide angles into two equal parts. This can be useful for solving problems involving triangles, quadrilaterals, and other polygons.
What are some real-world applications of perpendicular lines?
Perpendicular lines are used in a variety of real-world applications, including architecture, engineering, and construction. For example, perpendicular lines are used to ensure that buildings are level and stable.